ディンキン図形

索引トップ用語の索引ランキングカテゴリー

出典: フリー百科事典『ウィキペディア（Wikipedia）』 (2021/04/17 01:16 UTC 版)

図形の他の写像

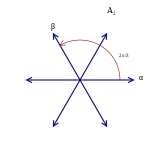A₂ ルート系	G₂ ルート系

図形のいくつかの追加の写像は以下に詳述するように意味のある解釈を持つ。しかしながら、ルート系のすべての写像が図形の写像として生じるわけではない^[10]。

例えば、A₂ の G₂ へのルート系の包含は2つあり、1つは6つの長いルートへの、もう1つは6つの短いルートへの写像である。しかしながら、G₂ 図形の2つの頂点は、1つは長いルートに、もう1つは短いルートに対応するが、A₂ 図形の頂点は等しい長さのルートに対応するから、ルート系のこの写像は図形の写像としては表せない。

ルート系のある包含は1つの図形の別の図形の誘導部分グラフ、すなわち「頂点は部分集合で、辺はそれらの間の全て」と表せる。なぜならば、ディンキン図形から頂点を取り除くことはルート系から単純ルートを取り除くことに対応し、これは階数が 1 小さいルート系になるからである。対照的に、頂点は変えずに辺を取り除くこと（あるいは辺の重複度を変えること）はルート間の角度を変えることに対応し、これはルート系全体を変えずにはできない。したがって、意味があるように頂点を取り除くことはできるが、辺ではできない。連結図形から頂点を取り除くと、頂点が葉ならば連結図形（単純リー環）になり、あるいは、2つか3つの成分からなる不連結図形（半単純だが単純でないリー環）になるかもしれない（後者は $D n$ と $E n$ に対して）。リー環のレベルでは、これらの包含は部分リー環に対応する。

極大部分グラフは以下のようである；図形の自己同型によって関連する部分グラフは "conjugate" とラベル付けられている：

A_n+1: A_n, in 2 conjugate ways.
B_n+1: A_n, B_n.
C_n+1: A_n, C_n.
D_n+1: A_n (2 conjugate ways), D_n.
E_n+1: A_n, D_n, E_n.
- For E₆, two of these coincide: $\mathrm {D} _{5}\cong \mathrm {E} _{5}$ and are conjugate.
F₄: B₃, C₃.
G₂: A₁, in 2 non-conjugate ways (as a long root or a short root).

最後に、図式の双対性は、存在すれば、矢印の向きの反転に対応する^[10]： $B n$ と $C n$ は双対であり、 $F 4$ や $G 2$ や simply-laced ADE 図形は自己双対である。

脚注

注

^ この節では明確にするために一般のクラスを「コクセター・ディンキン図形」ではなく「コクセター図形」と呼ぶ。混乱の可能性が大きく、また簡潔のためである。
^ Stekloshchik の矢印の向きはこの記事とは逆であることに注意。

出典

^ Baez, John (April 13, 1998), This Week's Finds in Mathematical Physics (Week 119)
^ Fulton & Harris 1991, Proposition D.40.
^ ^a ^b ^c Outer automorphisms of simple Lie Algebras
^ & Humphreys 1972, Section 16.5.
^ Jacobson 1971, section 7.
^ Algebraic geometry and number theory: in honor of Vladimir Drinfeld's 50th Birthday, edited by Victor Ginzburg, p. 47, section 3.6: Cluster folding
^ ^a ^b Folding by Automorphisms, John Stembridge, 4pp., 79K, 20 August 2008, Other Articles by John Stembridge
^ これらの foldings の絵と文献については次を参照：(Stekolshchik 2008, p. 102, remark 5.4).
^ Zuber, Jean-Bernard. Generalized Dynkin diagrams and root systems and their folding. pp. 28–30.
^ ^a ^b Transformations of Dynkin Diagrams, John Armstrong, March 5, 2010
^ ^a ^b (Knapp 2002, p. 758)
^ ^a ^b ^c Why are the Dynkin diagrams E6, E7 and E8 always drawn the way they are drawn?
^ Notes on Coxeter Transformations and the McKay correspondence, Rafael Stekolshchik, 2005, Section 2.1 The Cartan matrix and its Tits form p. 27. [1]
^ 例えば次を参照: Reflection groups and Coxeter groups, by James E. Humphreys, p. 96
^ [2] Infinite dimensional Lie algebras, Victor Kac
^ Carbone, L, Chung, S, Cobbs, C, McRae, R, Nandi, D, Naqvi, Y, and Penta, D: Classification of hyperbolic Dynkin diagrams, root lengths and Weyl group orbits, J. Phys. A: Math. Theor. 43 155209, 2010, arXiv:1003.0564
^ The symmetry of M-theories, Francois Englert, Laurent Houart, Anne Taormina and Peter West, 2003